Keyah Math Study 11, Level 3
Mathematical Content: of a circle, geometry, volume of a sphere, basic algebra, formulae for gravitational attraction
 Size, Mass and Density of the Earth:
Size, Mass and Density of the Earth:
This study has two parts. In the first part you will estimated the circumference, radius and volume of the Earth. In the second part you will determine the mass and density of the Earth. This module combines the material from KM#4 and KM#9
Part 1: Estimating the Circumference of the Earth
The goal of this study is to learn how Eratosthenes made the first close estimate of the circumference of the Earth and then use his estimate to compute its radius and volume.
Warm up question:
Without leaving this country, how could you figure out how far it is all the way around the World?
Introduction
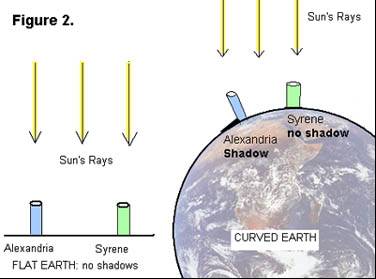
Around 250 BC, at noon on the day of the summer solstice (when the sun is at its highest point in the Northern Hemisphere) in Syrene, Egypt, sunlight filled the vertical shaft of a well; this indicates that the sun is directly overhead, so a vertical pole would cast no shadow. Eratosthenes, who lived in Alexandria, heard of this from a traveler. So on the same day, different year, he noticed that in Alexandria, some 800 kilometers (km) away, a vertical pole cast a shadow. From these observations, he made two deductions:
A. the earth is curved;
B. found the first estimate for the circumference of the Earth.
The Earth is Spherical
He measured the angle made by the pole and a line joining the tip of the shadow and the top of the pole (see Figure 1) and found the angle to be about 7o. Then he assumed that light rays from the sun to the Earth were essentially parallel since the sun was so far away and the Earth was so small relative to the sun. From this, and his observations in Alexandria and Syrene, he concluded that the Earth must be curved (see Figure 2), and therefore must be spherical.
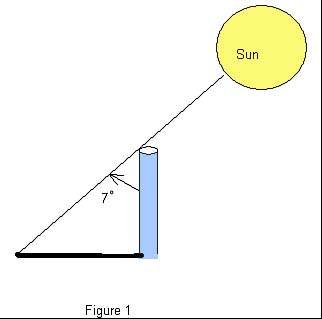
Using Math to Find the Circumference of the Earth
Next, he used all this information to obtain the first nearly accurate estimation of the circumference of the Earth. Here’s how:
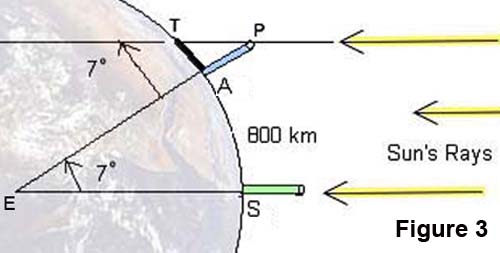
In the (not-to-scale) Figure 3:
A denotes the base of the pole in Alexandria;
S the base of a pole in Syrene;
T the tip of the shadow cast by the pole in Alexandria;
P the top of the same pole;
E the center of the Earth.
Angle APT was measured to be 7o, so by Euclidean geometry interior angles 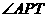 and
and 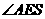 are equal, thus angle
are equal, thus angle 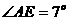 .
.
There are 360o in a complete circle, so the portion of the circumference of the Earth between A and S is
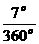 , which is approximately
, which is approximately  (or,
(or, 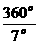 is approximately 50).
is approximately 50).
The distance from Alexandria to Syrene is 800 km, so he concluded that the circumference of the Earth must be
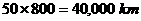 !
!
This estimate is very close to modern accurate measurements, so Eratosthenes gets credit for the first calculation of the size of the Earth.
We can get a slightly different answer if we compute more accurately:  , so if we multiply
, so if we multiply
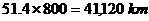


 , describes the force with which two objects attract each other.
, describes the force with which two objects attract each other.  and
and  denote the masses of the two objects
denote the masses of the two objects 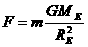
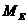 is the mass of the earth and
is the mass of the earth and  is the radius of the earth.
is the radius of the earth. 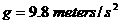 and we have
and we have 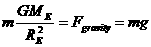 ,which simplifies to our basic equation
,which simplifies to our basic equation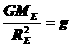 .
. to estimate the mass, volume and density of the earth.
to estimate the mass, volume and density of the earth.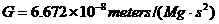
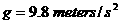
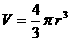
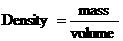
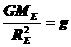 .
.